A) Knuckle Joint Design Procedure

Before going into detailed steps to design the dimensions of the Knuckle joint, it is essential to get oriented with all its components and their functions. Here is the diagram showing the exploded view of Knuckle Joint.

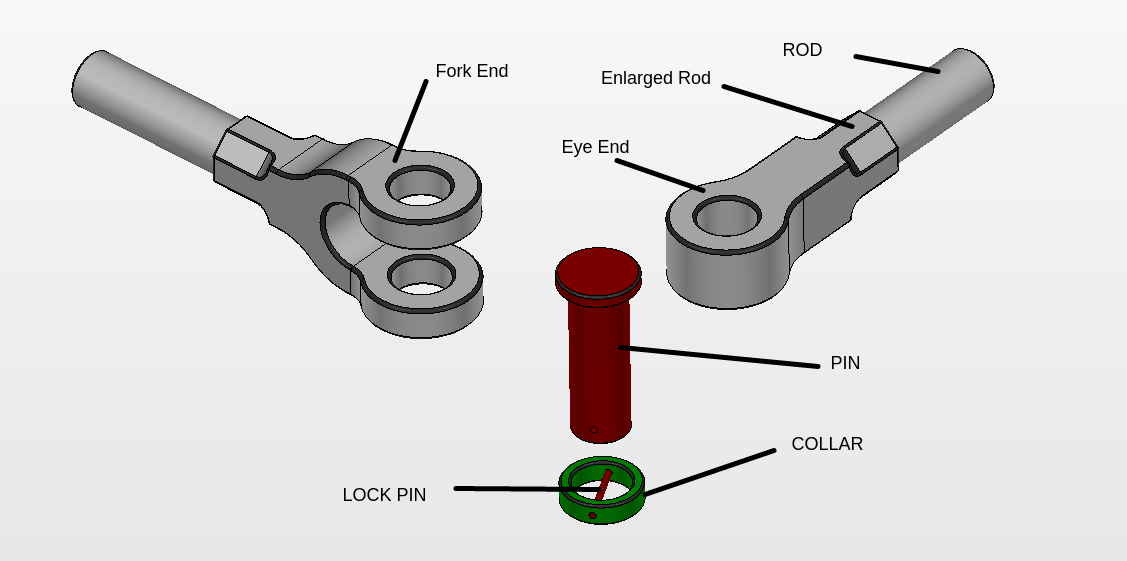
Knuckle Joint Asssembly Exploded View

As seen in the assembly the Knuckle joint has main four parts

Rods {Which are to be connected by joint }
Single eye {Modified rod for assembly}
Double eye or Forked end {Modified rod for assembly}
Pin {Connects the two rods}
Collar {to keep the pin in position}
Split pin or taper pin {Not in diagram} {to prevent sliding away of pin}

Assembly Drawing and Notations used in Knuckle Joint

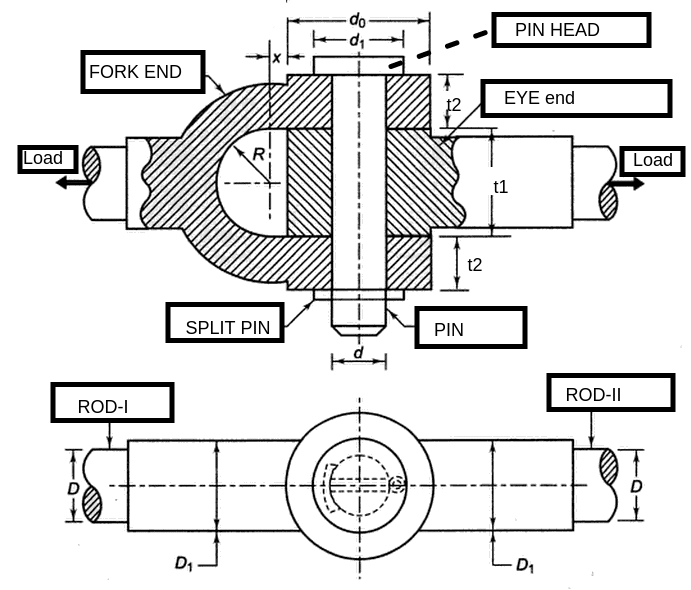
Knuckle Joint Crosssectional View

Notations used in design :

P = Tension in rod ( Load on the joint)

D = Diameter of rod

D₁= Enlarged diameter of rod

d = Diameter of pin

d₁ = Diameter of pin head

d₀ = Outer diameter of eye or fork

t1 = thickness of eye end

t2= thickness of forked end (double eye)

x= distance of the Centre of fork radius R from the eye

STEPS TO DESIGN KNUCKLE JOINT

Step 1 : Design of Rods (D,D₁)

Tensile failure of rod

Knuckle Joint Tensile failure of rod

Using basic strength equation

Load = Stress * Area

$P = (Area \quad Resisting \quad Tension) \times (Allowable \quad Stress)$

$P= \frac{\pi}{4} \times D^2 \times \sigma_t$ From This equation Diameter ‘D’ of rod can be found

Empirical relations

Using Empirical relations the enlarged diameter of rod D₁ is determined

$D_1 =1.1 \times D$

Step 2 : Decide the thickness of eye end and forked end (t₁,t₂)

Empirical relations

Both these dimensions are decided on the basis of empirical relations,

t₁= 1.25 D and t₂= 0.75 D

Step 3 : Decide the dimensions of pin (d,d₁)

Double shear Failure

The pin may get sheared off into three pieces as shown below, since the pin breaks at two places it is called double shear. Both areas are taken as resisting areas.

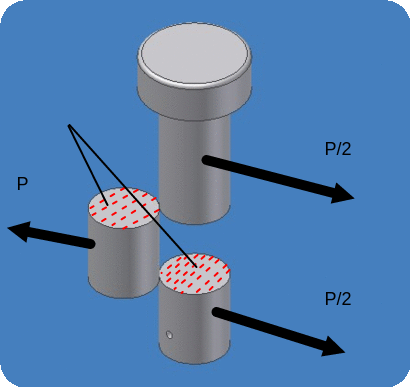
Knuckle Joint Double shear Failure of pin

Using basic strength equation

Load = Stress * Area

$P = (Area \quad Resisting \quad Tension) \times (Allowable \quad Stress)$

$P= {\color{Red} 2} \times \frac{\pi}{4} \times d^2 \times \sigma_s$

Note that 2 is because of double shear ...From This equation Diameter ‘d’ of pin can be found. But since the pin is also subjected to bending one more diameter of pin on the basis of bending is determined and the bigger of both is taken as the final size of pin

Bending failure of pin

The diameter on the basis of bending is determined using the following formula,

$d =\sqrt[3]{\frac{32}{\pi \sigma_b} \times \frac{P}{2}[\frac{t_1}{4}+\frac{t_2}{3}]}$ …..Calculate d from this formula

{ Discussion on how this formula is obtained is in the theory question and answer section of knuckle joint }

Empirical relation for pin head diameter

Since pin head is not subjected to any stress, its diameter is simply decided on the basis of proportionality, (it is taken 50% more than that of pin diameter )

d₁=1.5 d

Step 4 : Check Stresses in Eye end

Empirical relation for outside diameter of eye and fork

d₀=2d

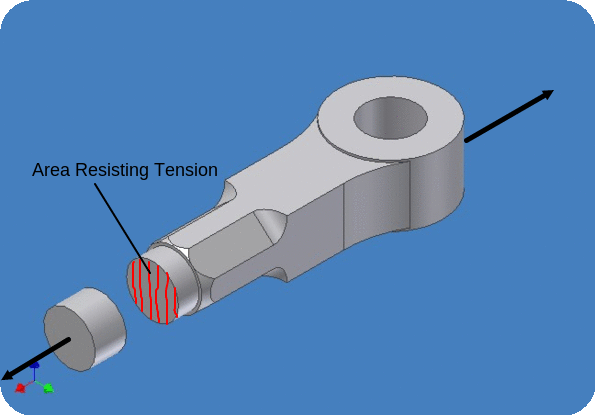
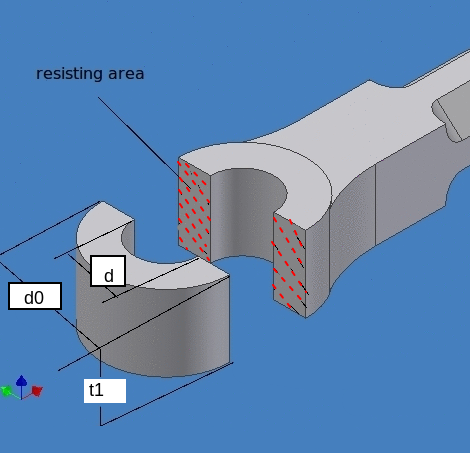
Tensile failure of eye end

The single eye may fail in tension as shown below { please note that when the plane of failure is perpendicular to the direction of force then the failure is either tensile or compressive}

Knuckle joint Tensile failure of single eye

Using the basic equation for stress

$P = (Area \quad Resisting \quad Tension) \times (Allowable \quad Stress)$

$P= (d_0-d)t_1 \times \sigma_t$ ………….Using this equation find the value of $\sigma_t$ and check if it is less than allowable value for design to be safe.

{Note that area resisting the tension is rectangular one and not circular so its area is length time height total length is (d0-d) and height is t1.

Shear failure of eye end

The single eye may fail in shear as shown below { please note that when the plane of failure is parallel to the direction of force then the failure is Shear failure}

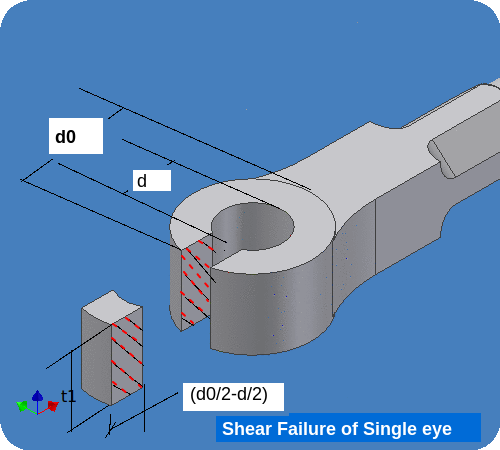
Knuckle Joint Shear failure of single eye

Using the basic equation for stress

$P = (Area \quad Resisting \quad Tension) \times (Allowable \quad Stress)$

$P= 2 \times (\frac {d_0}{2}- \frac{d}{2})t_1 \times \sigma_s$ simplifying this equation we get

$P= ({d_0}- d) \times t_1 \times \sigma_s$ ………….Using this equation find the value of $\sigma_s$ and check if it is less than allowable value for design to be safe.

Crushing Failure of eye end

The single eye is also subjected to Crushing between pin and inner face of single eye. In case of crushing failure since the area is curved we take the projected (area which would be visible in drawing) of the cylindrical area. As we know that a cylinder appears as a rectangle in projection, hence the area will be diameter times the height of cylinder. This area is illustrated below,

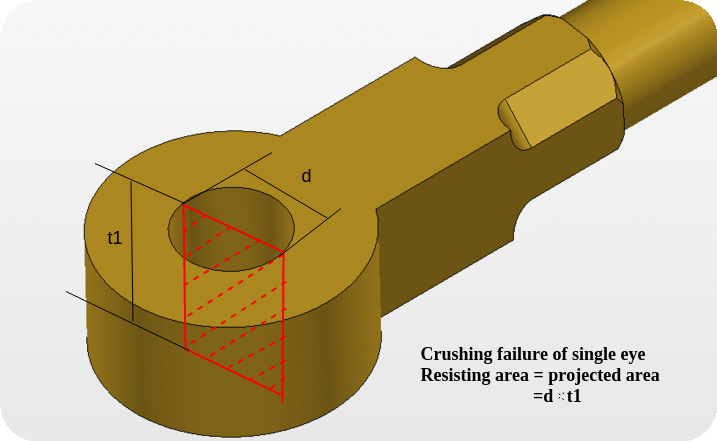
Knuckle joint Crushing failure of single eye

Using the basic equation for stress

$P = (Area \quad Resisting \quad Tension) \times (Allowable \quad Stress)$

$P= d \times t_1 \times \sigma_c$ ………….Using this equation find the value of $\sigma_c$ and check if it is less than allowable value for design to be safe.

Step 5 : Check Stresses fork end

Fork end is also subjected to same failures as that of eye end, the only difference is that it has two eyes. So we get the same equations except multiplied by 2.

The equations for tensile, shear and crushing failures are given below

Tensile failure of fork end

$P={\color{Red} 2} \times (d_0-d) {\color{Red} t_2} \times \sigma_t$ {see the changes highlighted in red from the equation of single eye} Get the value of induced tensile stress from this equation and confirm that it is below allowable tensile stress.

Shear failure of fork end

$P={\color{Red} 2} \times (d_0-d) {\color{Red} t_2} \times \sigma_s$ {see the changes highlighted in red from the equation of single eye} Get the value of induced shear stress from this equation and confirm that it is below allowable shear stress.

Crushing failure of fork end

$P={\color{Red} 2} \times d \times {\color{Red} t_2} \times \sigma_s$ {see the changes highlighted in red from the equation of single eye} Get the value of induced crushing stress from this equation and confirm that it is below allowable crushing stress.

Summary of Design Procedure in Tabular form

{ Student preparing for exam find the tabular form very handy and easy to remember the formulae }

Step

Failure

Equation

To find

Step 1 : Design of Rods (D,D₁)

Tensile

Empirical

$P= \frac{\pi}{4} \times D^2 \times \sigma_t$

$D_1 =1.1 \times D$

D1=

Step 2 : Decide the thickness of eye end and forked end (t₁,t₂)

Empirical relations

t₁= 1.25 D

t₂= 0.75 D

t1 =

t2 =

Step 3 : Decide the dimensions of pin (d,d₁)

Double shear

Bending failure

Empirical

$P= {\color{Red} 2} \times \frac{\pi}{4} \times d^2 \times \sigma_s$

$d =\sqrt[3]{\frac{32}{\pi \sigma_b} \times \frac{P}{2}[\frac{t_1}{4}+\frac{t_2}{3}]}$

d₁=1.5 d

d =

{Choose higher value of both}

Step 4 : Check Stresses in Eye end

Empirical

Tensile

Shear

Crushing

d₀=2d

$P= (d_0-d)t_1 \times \sigma_t$

$P= ({d_0}- d) \times t_1 \times \sigma_s$

$P= d \times t_1 \times \sigma_c$

d₀=

check $\sigma_t$

check $\sigma_s$

check $\sigma_c$

Step 5 : Check Stresses fork end

Tensile

Shear

Crushing

$P={\color{Red} 2} \times (d_0-d) {\color{Red} t_2} \times \sigma_t$

$P={\color{Red} 2} \times (d_0-d) {\color{Red} t_2} \times \sigma_s$

$P={\color{Red} 2} \times d \times {\color{Red} t_2} \times \sigma_s$

check $\sigma_t$

check $\sigma_s$

check $\sigma_c$

NUMERICAL PROBLEMS

1) It is required to design a Knuckle joint to connect two circular rods which are subjected to an axial tensile load of 50 kN. the material used for joints is 50c8, having  yield point stress 400 N/mm2. taking factor of safety as 5, find the all dimensions of Knuckle joint.


2) A Knuckle joint carries  an axial load of 100 kN.  it connects two circular rods which are made up of material 40c8, having  yield strength 360 N/mm2.  using factor of safety 4 design and draw the Knuckle joint.  Show all important dimensions in the final drawing.


3) Design a Knuckle joint to transmit a load of 50 kN, assume permissible stresses as 40 N/mm² in 10 size 40 N/mm² in shear and 80 N/mm² in compression.Also draw neat dimensioned sketch of Knuckle joint showing all important dimensions designed.


4) designer Knuckle joint subjected to a pull of 30000N.  assume permissible stresses as below,

 allowable tensile stress 80 N/mm²

allowable shear stress  60 N/mm²

 allowable crushing stress  120 N/mm²

5) A knuckle joint is required to transmit a load of 150 kN. the rod end, fork end  and pin are made up of same material.  The design stresses may be taken as

 allowable tensile stress 75 N/mm²

 allowable shear stress 60 N/mm²

 allowable crushing stress 150 N/mm²

6) It is required to design a Knuckle joint for a tie rod of circular cross section,  the joint is subjected to maximum Pull of 70 kN.  the ultimate strength of the material of the rod against tearings  is 420  Mpa . the ultimate tensile and shearing strength of pin material or 510  Mpa and 400 Mpa respectively. Design the single eye, double eye  and pin. Take factor of safety is equal to 6

7)Design a knuckle joint required to connect two  steel bars subjected to a tensile load of 25 kN. The allowable stresses are 
75 MPa in tension, 
60 MPa in shear and 
100 MPa in crushing. Also draw the neat dimensioned sketch of the joint showing all important obtained dimensions.

8) Two MS rods are to be connected by a knuckle joint, load it has to transmit  is  120 kN. Design the joint assuming that the working stresses for both the pin and rod materials to be,

85 MPa in tension,

70 MPa in shear and

140MPa in crushing.

You are here

Knuckle Joint : Design Procedure,Problems and Questions

A) Knuckle Joint Design Procedure

Notations used in design :

STEPS TO DESIGN KNUCKLE JOINT

Step 1 : Design of Rods (D,D1)

Tensile failure of rod

Empirical relations

Step 2 : Decide the thickness of eye end and forked end (t1,t2)

Empirical relations

Step 3 : Decide the dimensions of pin (d,d1)

Double shear Failure

Load = Stress * Area

Bending failure of pin

Empirical relation for pin head diameter

Step 4 : Check Stresses in Eye end

Empirical relation for outside diameter of eye and fork

Tensile failure of eye end

Shear failure of eye end

Crushing Failure of eye end

Step 5 : Check Stresses fork end

Tensile failure of fork end

Shear failure of fork end

Crushing failure of fork end

Summary of Design Procedure in Tabular form

Step

Failure

Equation

To find

Step 5 : Check Stresses fork end

NUMERICAL PROBLEMS

C) Theory Questions and answers on Knuckle joint

Theory questions are available here - Theory Questions Link

D) Objective type Questions and answers on Knuckle joint

Links to other Topics in Machine design I

Our Sister Sites

Step 1 : Design of Rods (D,D₁)

Step 2 : Decide the thickness of eye end and forked end (t₁,t₂)

Step 3 : Decide the dimensions of pin (d,d₁)