Two parallel shafts whose centre lines are 4.8 m apart are
connected by open belt drive. The diameter of larger pulley is
1.5 m and that of smaller pulley 1 m. The initial tension in
the belt when stationary is 3 kN. The mass of the belt is
1.5 kg/m length. The coeff of friction between belt and pulley
is 0.3. Taking centrifugal tension in to account. Calculate
power transmitted when smaller pulley rotates at 400 rpm
Two parallel shafts whose centre lines are 4.8 m apart are connected by open belt
drive. The diameter of larger pulley is 1.5 m and that of smaller pulley 1 m. the initial
tension in the belt when stationary is 3 KN. The mass of the belt is 1.5 Kg/m length.
The coefficient of friction between belt and pulley is 0.3. Taking centrifugal tension
in to account, calculate power transmitted when smaller pulley rotates at 400 rpm.
Given Data:
Open Belt Drive:
Where,
C = 4.8 m
D 1 = 1.5 m
D 2 = 1 m
N 2 = 400 rpm
3
Ti = 3 KN = 3 x 10 N
m = 1.5 Kg/m length
μ = 0.3
Considering Centrifugal Tension (Tc) =mV 2
[1] We know that, Velocity (V) of the Open Belt Drive;
[2] Centrifugal Tension in Belt (Tc),
(T c ) =mV 2 = 1.5 x (21) 2 = 661.5 N
[3] We know that initial tension in Belt (T i ) as,
Let, T 1 = Tension in Tight Side (N)
T 2 = Tension in Slack Side (N)
[3] We know that initial tension in Belt (T i ) as,
Let, T 1 = Tension in Tight Side (N)
T 2 = Tension in Slack Side (N)
T i =
3000 x 2 = T 1 + T 2 + 2 x (661.5)
T 1 + T 2 = 4677 N ....................................Eq. [1]
[4] For an Open Belt Drive,
So, angle of lap on the smaller pulley is;
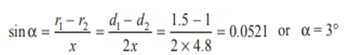
marks:
- Log in to post comments
