Theory

Applications- Automobiles like scooters, motorcycles, textile and paper industries, machine tools

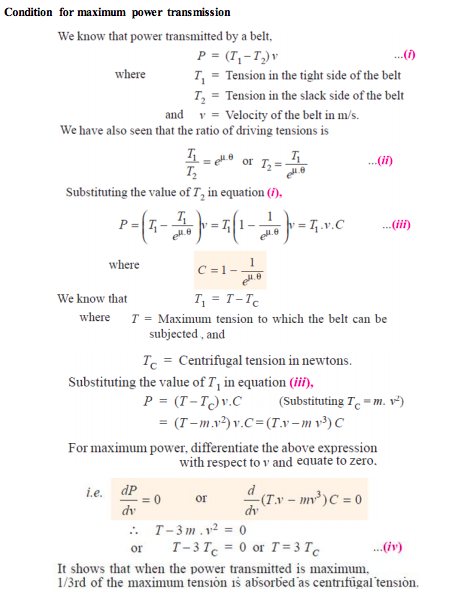
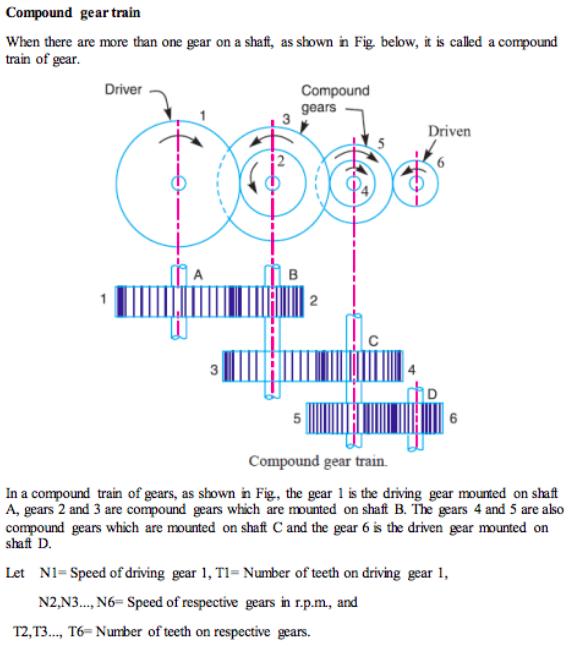
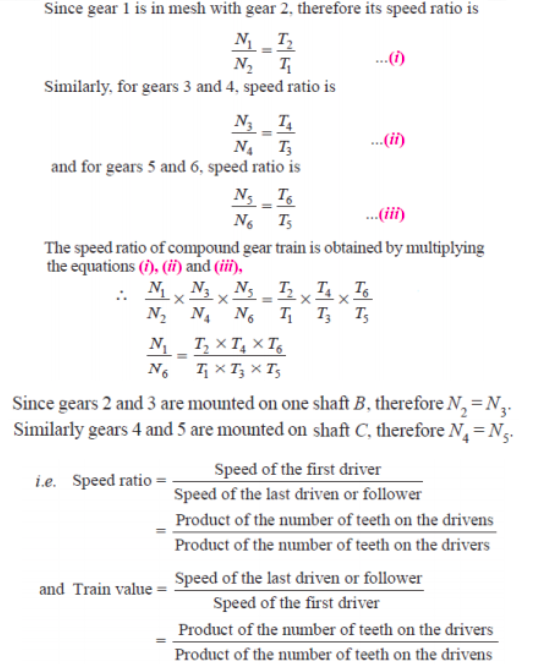
Linear Velocity: It may be defined as the rate of change of linear displacement of a body with respect to the time. Since velocity is always expressed in a particular direction, therefore it is a vector quantity. Mathematically, linear velocity, v = ds/dt

Angular Velocity: It may be defined as the rate of change of angular displacement with respect to time. It is usually expressed by a Greek letter ɷ (omega). Mathematically, angular velocity, ɷ = dƟ /dt

i. Prime circle: It is the smallest circle that can be drawn from the centre of the cam and tangent to the pitch curve. For a knife edge and a flat face follower, the prime circle and the base circle are identical. For a roller follower, the prime circle is larger than the base circle by the radius of the roller.

ii. Pitch circle: It is a circle drawn from the centre of the cam through the pitch points.

Classification of cam:

1. Radial or disc cam: In radial cams, the follower reciprocates or oscillates in a direction perpendicular to the cam axis. The cams as shown in above Fig. are all radial cams.

2. Cylindrical cam: In cylindrical cams, the follower reciprocates or oscillates in a direction parallel to the cam axis. The follower rides in a groove at its cylindrical surface. A cylindrical grooved cam with a reciprocating and an oscillating follower is shown in Fig. below (a) and (b) respectively.

Sliding pair :

When the two elements of a pair are connected in such a way that one can only slide relative to the other, the pair is known as a sliding pair. The piston and cylinder, cross-head and guides of a reciprocating steam engine, ram and its guides in shaper, tail stock on the lathe bed etc. are the examples of a sliding pair. A little consideration will show that a sliding pair has a completely constrained motion.

Inversions of Double Slider Crank Chain :

1. Elliptical trammels.

2. Scotch yoke mechanism.

3. Oldham’s coupling.